Soil Erosion Risk Calculator:Calculators for Students, Engineers & Researchers:free Online Tool

Soil Erosion Risk Calculator

Slope (in degrees): Soil Type: Sandy Loam Clay Land Cover: Forest Grassland Cropland Rainfall Intensity (in mm/h):

Soil Erosion Risk:

Pesticide Dosage Calculator:Calculators for Students, Engineers & Researchers:free Online Tool

Pesticide Dosage Calculator

Pest Type: Insect Fungus Weed

Crop Stage: Seedling Vegetative Flowering Fruiting

Desired Control Level: Low Medium High

Livestock Feed Ration Calculator:Calculators for Students, Engineers & Researchers:free Online Tool

Livestock Feed Ration Calculator

Livestock Type: Cattle Sheep Goat Weight (in kg): Protein Requirement (%): Energy Requirement (MJ/kg): Available Feed Ingredients:

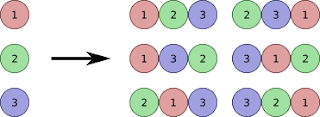
Permutation and Combination Calculator:Engineering & Science Calculators: Free Online Tools

Definition: Permutation and combination are two fundamental concepts in probability and counting problems. A combination is a selection of items from a set, regardless of order.

Click the Translate button(see right) on this post to set your Own Language to understand more perfectly!!

Permutation and Combination Calculator

n:
r:

Continue Definition:

Permutation and combination are two fundamental concepts in probability and counting problems. Here's a breakdown of each concept with supporting equations and solved examples:

Permutation:

A permutation is an arrangement of a set of distinct objects in a specific order. The order matters, so "ABC" is considered different from "CBA."

Formula:

The number of permutations of n distinct objects (nPr) is calculated using the following formula:

nPr = n! / (n - r)!

where:

n: Total number of objects

r: Number of objects chosen for arrangement

!: Factorial (denoted by an exclamation mark), which represents the product of all positive integers less than or equal to a number (e.g., 4! = 4 * 3 * 2 * 1)

Example:

Imagine you have 3 friends (Alice, Bob, and Charlie) and need to choose 2 of them for a movie night. How many different combinations of 2 friends can you have (considering the order matters)?

Here, n (total objects) = 3 and r (objects chosen) = 2.

Using the formula:

nPr = 3! / (3 - 2)! = 3 * 2 * 1 / 1 = 6

Therefore, there are 6 different ways you can choose 2 friends for the movie night in a specific order (e.g., Alice and Bob, Bob and Alice, etc.).

Combination:

A combination is a selection of items from a set, regardless of order. So, "ABC" and "CBA" would be considered the same combination in this case.

Formula:

The number of combinations of n distinct objects taken r at a time (nCr) is calculated using the following formula:

nCr = n! / (r! * (n - r)!)

where:

n and r are the same as in permutations.

Example:

Let's say you have 4 different flavors of ice cream (Vanilla, Chocolate, Strawberry, and Mango) and want to choose 2 for a sundae. How many different combinations of flavors can you have (regardless of the order you add them)?

Here, n (total objects) = 4 and r (objects chosen) = 2.

Using the formula:

nCr = 4! / (2! * (4 - 2)!) = 4 * 3 / (2 * 2) = 6

Therefore, there are 6 different combinations of ice cream flavors you can choose for the sundae, even though the order of adding them doesn't matter (Vanilla-Chocolate is the same as Chocolate-Vanilla).

Key Differences:

The main difference lies in whether order matters. Permutations consider order, while combinations do not. This is reflected in the formulas:

Permutation formula has (n - r)! in the denominator.

Combination formula has an additional r! in the denominator compared to the permutation formula.

In conclusion:

Understanding permutations and combinations is crucial for solving various counting problems. By using the appropriate formula and considering the importance of order, you can accurately determine the number of possible arrangements or selections for a given scenario.

let's start with the mathematical equations and notation for permutations and combinations:

**Permutations:**

Permutations are arrangements of objects in a certain order. The formula to calculate permutations is:

[ P(n, r) = frac{n!}/{(n - r)!} ]

Where:

( P(n, r) ) represents the number of permutations of ( n ) objects taken ( r ) at a time.

( n! ) denotes the factorial of ( n ), which is the product of all positive integers up to ( n ).

( (n - r)! ) denotes the factorial of ( n - r ).

**Combinations:**

Combinations are selections of objects without considering the order. The formula to calculate combinations is:

[ C(n, r) = frac{n!}/{r!(n - r)!} ]

Where:

( C(n, r) ) represents the number of combinations of ( n ) objects taken ( r ) at a time.

( n! ) denotes the factorial of ( n ).

( r! ) denotes the factorial of ( r ).

( (n - r)! ) denotes the factorial of ( n - r ).

**Questions with solving hints:**

1. **Question:** In how many ways can you arrange 5 books on a shelf?

**Hint:** This is a permutation problem. You want to calculate ( P(5, 5) ).

2. **Question:** How many different 3-letter codes can you make using the letters A, B, C, D, and E?

**Hint:** This is also a permutation problem. Calculate ( P(5, 3) ).

3. **Question:** If a pizza parlor offers 8 toppings and you can choose 3 toppings for your pizza, how many different combinations of toppings are possible?

**Hint:** This is a combination problem. Calculate ( C(8, 3) ).

4. **Question:** A committee of 4 people needs to be formed from a group of 10 candidates. How many different committees can be formed?

**Hint:** This is also a combination problem. Calculate ( C(10, 4) ).

5. **Question:** How many different 5-card hands can be dealt from a standard deck of 52 cards?

**Hint:** This is a combination problem. Calculate ( C(52, 5) ).

**Ways to utilize permutation & combination calculation to earn money:**

1. **Event Planning:** Event planners use combinations to arrange seating plans, menu options, and other arrangements for events like weddings, conferences, and parties.

2. **Stock Trading:** Understanding permutations and combinations can help in a

nalyzing different investment portfolios and predicting stock movements.

3. **Marketing Strategies:** Marketers use permutations and combinations to analyze customer data and develop targeted advertising campaigns, product bundling strategies, and pricing models.

4. **Gaming and Gambling:** Casinos and gaming companies use permutation and combination calculations to design games, set odds, and analyze player behavior.

5. **Software Development:** Permutations and combinations are used in software development for tasks like generating unique codes, passwords, and cryptographic keys.

Do YOU Want To Earn Money In Various Ways, Click The Link & Explore Your Field of Interest!!!

Integral Calculator:Calculators for Students, Engineers & Researchers:free Online Tool

Integral Calculator

Function:

Second Order Differential Equation Calculator:Calculators for Students, Engineers & Researchers:free Online Tool

Second Order Differential Equation Calculator

Coefficient a:

Coefficient b:

Coefficient c:

Result:

Algebraic Calculator:Calculators for Students, Engineers & Researchers:free Online Tool

Algebraic Calculator

Geometry Calculator:Calculators for Students, Engineers & Researchers:free Online Tool

Geometry Calculator

Enter the length:

Enter the width:

Trigonometric Calculator:Calculators for Students, Engineers & Researchers:free Online Tool

Definition: A trigonometric calculator is a tool used to compute the values of trigonometric functions for a given angle. Trigonometry itself is a branch of mathematics that deals with the relationship between angles and side lengths in triangles. The most common trigonometric functions are sine (sin), cosine (cos), and tangent (tan).The rest trigonometric functions are Cosec, Sec, & cot. And are the inverse of sin, cos & tan respectly.

Click the Translate button(see right) on this post to set your Own Language to understand more perfectly!!

Trigonometric Calculator

Enter the angle in degrees:

Continue Definition:

A trigonometric calculator is a tool used to compute the values of trigonometric functions for a given angle. Trigonometry itself is a branch of mathematics that deals with the relationship between angles and side lengths in triangles. The most common trigonometric functions are sine (sin), cosine (cos), and tangent (tan)

.

Here's a breakdown of these functions with supporting equations and an example:

Sine (sin): Sine is defined as the ratio of the opposite side (side facing the right angle) to the hypotenuse (longest side) of a right triangle.

Equation: sin(θ) = opposite / hypotenuse

Cosine (cos): Cosine is defined as the ratio of the adjacent side (side next to the angle) to the hypotenuse of a right triangle.

Equation: cos(θ) = adjacent / hypotenuse

Tangent (tan): Tangent is defined as the ratio of the opposite side to the adjacent side of a right triangle.

Equation: tan(θ) = opposite / adjacent

Where, a=opposite, b=Hypotenuse, & c=Adjacent or base.

Example:

Let's consider a right triangle with an angle of 30 degrees (θ = 30°). The opposite side has a length of 6 units, and the hypotenuse has a length of 10 units. We don't have the value of the adjacent side yet.

Using the sine function: sin(30°) = 6 / 10 = 3/5

Using the cosine function (let's find the adjacent side first): cos(30°) = adjacent / 10. We know sin(30°) = 1/2 (using the unit circle or trigonometric tables), so cos(30°) = √(1 - sin²(30°)) = √(1 - (3/5)²) = √(16/25) = 4/5. Therefore, adjacent = cos(30°) * 10 = 4 * 10 / 5 = 8.

Using the tangent function: tan(30°) = 6 / 8 = 3/4

A trigonometric calculator can take the angle value (30° in this case) and the type of function (sine, cosine, or tangent) as input and provide the corresponding trigonometric value (3/5 for sine, 4/5 for cosine, and 3/4 for tangent) as output. Many calculators also offer functions like inverse sine (arcsin), inverse cosine (arccos), and inverse tangent (arctan), which allow you to find the angle for a given trigonometric value.

How to EARN MONEY using Trigonometric Calculator in our practical Life???

Trigonometry has a wide range of applications in practical life that can indirectly help you earn money.

Here are some specific examples:

1. Construction and Surveying:

Land surveying: Trigonometric calculations are crucial for land surveyors to measure distances and angles on a property. They use functions like sine, cosine, and tangent to determine property lines, plot elevations, and calculate areas for construction purposes.

Building design and construction: Trigonometry helps architects and engineers design structures that are stable and functional. They use trigonometry to calculate roof pitches, determine load-bearing capacities, and ensure proper placement of beams and columns. If you're skilled in these areas, your understanding of trigonometry can make you a valuable asset and potentially lead to higher paying jobs or freelance opportunities.

2. Engineering Fields:

Civil Engineering: Similar to construction, civil engineers rely on trigonometry for designing roads, bridges, dams, and other infrastructure projects. They use it to calculate forces, angles, and stress distribution to ensure the stability of these structures.

Electrical Engineering: Trigonometry plays a role in analyzing and designing electrical circuits. It helps engineers calculate voltage, current, and impedance in AC circuits, which is essential for efficient power transmission and distribution. Having strong trigonometric skills can make you a more qualified candidate in these engineering fields, potentially increasing your earning potential.

3. Other Fields:

Animation and Video Game Design: Trigonometry is used in 3D animation and video game design to create realistic movements and trajectories for characters and objects. By understanding angles and distances, animators can create natural-looking movements and game designers can program realistic physics.

Stock Trading (indirectly): While trigonometry isn't directly used for stock analysis, some technical indicators used in trading rely on trigonometric functions. However, a strong understanding of trigonometry isn't essential for success in stock trading.

Earning through Online Platforms:

Freelance Tutoring: If you have a strong understanding of trigonometry, you can offer online tutoring services to students struggling with the subject. Platforms like Upwork or Chegg Tutors allow you to connect with students and potentially earn money by teaching them trigonometric concepts.

Creating Educational Content: You can create online courses or educational content (like video tutorials or ebooks) explaining trigonometric concepts in a clear and engaging way. Platforms like Udemy or Skillshare allow you to sell your courses and potentially earn money by sharing your knowledge.

Remember, while trigonometry itself won't directly earn you money, having a strong understanding of it can be a valuable skill in various fields that do offer earning opportunities. It can make you a more qualified candidate for specific jobs or freelance work, or allow you to create educational content that others can pay for.

Do YOU Want To Earn Money In Various Ways, Click The Link & Explore Your Field of Interest!!!

Programmable Calculator:Calculators for Students, Engineers & Researchers:free Online Tool

Programmable Calculator

Complex Number Calculator:Calculators for Students, Engineers & Researchers:free Online Tool

Definition: The complex number system extends the familiar real numbers with a special element called the imaginary unit, denoted by "i". Here's the breakdown:Complex Number: Any number expressible in the form a + bi, where:a and b are real numbers. i is the imaginary unit, defined as i² = -1. Real part: The number "a" is called the real part of the complex number.Imaginary part: The number "b" times i is called the imaginary part of the complex number.

Click the Translate button(see right) on this post to set your Own Language to understand more perfectly!!

Complex Number Calculator

Real Part (a):
Imaginary Part (b):
Real Part (c):
Imaginary Part (d):

Result:

Continue Definition:

The complex number system extends the familiar real numbers with a special element called the imaginary unit, denoted by "i". Here's the breakdown: Complex Number: Any number expressible in the form a + bi, where: a and b are real numbers. i is the imaginary unit, defined as i² = -1. Real part: The number "a" is called the real part of the complex number. Imaginary part: The number "b" times i is called the imaginary part of the complex number.

Examples of complex numbers include:

3 + 5i (real part = 3, imaginary part = 5)

-2i (real part = 0, imaginary part = -2)

7 (This can be written as 7 + 0i, where the imaginary part is zero)

Properties of Complex Numbers:

Closure under addition, subtraction, multiplication, and division: You can perform these operations on complex numbers and still get a complex number as the result.

Commutative and associative properties: Addition and multiplication of complex numbers follow the commutative and associative properties, similar to real numbers.

Distributive property: The distributive property holds for complex numbers as well (a + b) * c = a * c + b * c

Inverting a Complex Number (Finding the reciprocal):

Inverting a complex number, similar to real numbers, means finding the number that multiplies to 1. The process involves using the fact (a + b) * (a - b) = a² - b².

Here's how it works:

Given complex number: z = a + bi (a and b are real numbers)

Denominator simplification: We want to avoid imaginary unit (i) in the denominator. So, we multiply both numerator and denominator by the denominator's complex conjugate, which is a - bi (same real part, opposite imaginary part).

Simplification: Apply the distributive property and the fact (a + b) * (a - b) = a² - b²

(a + bi) * (a - bi) = (a² - (bi²)) = a² + b² (i² = -1)

Therefore, the inverse of z (denoted as z⁻¹ ) is:

z⁻¹ = (a - bi) / (a² + b²)

Example:

Find the inverse of z = 3 + 2i

Apply the formula:

z⁻¹ = (3 - 2i) / (3² + 2²) = (3 - 2i) / 13

Separate real and imaginary parts:

z⁻¹ = (3/13) - (2/13)i

Therefore, the inverse of 3 + 2i is (3/13) - (2/13)i.

Application of the Complex number System:

Complex numbers might seem strange at first, but they have surprisingly practical applications in various fields:

Electrical Engineering: Analyzing AC circuits (Alternating Current) is a prime example. Complex numbers help represent voltage, current, and impedance (resistance to current flow). This simplifies calculations and allows engineers to predict circuit behavior.

Signal Processing: Complex numbers play a crucial role in analyzing signals, like sound and radio waves. They help decompose signals into their frequency components, which is essential for various applications like image and audio compression.

Control Systems: Complex numbers are used to design and analyze feedback control systems, which are found everywhere from airplanes to cruise control in cars. They help ensure systems remain stable and respond properly to external influences.

Computer Graphics: Complex numbers are used in 3D graphics to represent rotations and transformations of objects. This allows for smooth animations and realistic modeling of 3D scenes.

Quantum Mechanics: This branch of physics that deals with the world of atoms and subatomic particles heavily relies on complex numbers. They are used to describe the wave functions of particles, which are essential for understanding their behavior.

These are just a few examples, and complex numbers find applications in other areas like:

Fluid Mechanics: Describing fluid flow and wave propagation.

Fractals: Creating complex and self-similar geometric patterns.

Number Theory: Solving certain types of equations and understanding the properties of numbers.

So, next time you hear about complex numbers, remember they're not just abstract concepts. They are a powerful tool used in many real-world applications that shape our technological advancements and our understanding of the universe.

How to Earn Money By using the Knowledge of Complex Number System Calcultion?????

Here's how your knowledge of complex number calculations and utilization can translate into earning money:

Direct Applications:

Engineering Fields:

Electrical Engineer: If you pursue a career in electrical engineering, complex numbers become crucial for analyzing AC circuits, designing power systems, and understanding signal transmission.

Control Systems Engineer: In this field, you'd use complex numbers to design and analyze feedback control systems, ensuring stability in everything from airplanes to self-driving cars.

Communications Engineer: Complex numbers are essential for analyzing and designing communication systems, like radio and wireless networks.

Scientific Computing: Many scientific computing fields heavily rely on complex number manipulation. If you have strong programming skills alongside your complex number knowledge, you could work on simulations, data analysis, or developing software tools used in various scientific disciplines.

Indirect Applications:

Data Science and Machine Learning: While complex numbers might not be the core concept, they often appear in advanced data analysis and machine learning algorithms. A strong foundation in complex numbers would benefit you in understanding these algorithms and their applications in various fields.

Tutoring or Online Courses: If you have a knack for explaining complex concepts, you could offer tutoring services or create online courses specifically focused on complex numbers for engineering students or those interested in higher mathematics.

General Skills Development:

Problem-Solving and Analytical Skills: Complex number calculations require strong problem-solving and analytical thinking. These skills are highly valued across various industries and can help you excel in any analytical or technical role, even if it doesn't directly involve complex numbers.

Earning Potential:

The earning potential depends on your chosen career path, experience, and location. Engineering fields generally offer competitive salaries, with senior positions reaching well over $100,000 annually. Data science and scientific computing roles can also be quite lucrative depending on the industry and specific role.

Additional Tips:

Develop programming skills: Many employers look for engineers and scientists with strong programming skills alongside their mathematical background. Learning Python, MATLAB, or other relevant languages will significantly enhance your career prospects.

Consider postgraduate studies: A Master's degree in a relevant engineering field or scientific computing can further strengthen your expertise and open doors to higher-paying positions.

By leveraging your knowledge of complex numbers and developing complementary skills, you can position yourself for a successful career in various fields with excellent earning potential.

Do YOU Want To Earn Money In Various Ways, Click The Link & Explore Your Field of Interest!!!